Effective width of flanges <EM>[EC2 A§5.3.2.1]</EM>|

VOLUME A
The art of construction and detailing
Introduction
The structural frame
- Introduction
- Structural frame elements
  - Columns
  - Beams
  - Slabs
  - Staircases
  - Foundation
- STRUCTURAL FRAME LOADING
- BEHAVIOR OF THE STRUCTURAL FRAME
  - The behavior and reinforcement of a slab
  - Behavior and reinforcement of beams and columns
Construction methods
- The materials
- The moulds
- Thermal insulation of structural elements
- Concrete
- The steel
- Reinforcement specifications of antiseismic design
- Industrial stirrups - stirrup cages
- Standardized cross-sections of reinforced concrete elements
Reinforcement
- Columns
- Shear walls
- Composite elements
- Beams
- Slabs
- Staircases
- Foundation
Quantity surveying
- Estimation of the concrete’s quantity
- Estimation of the formworks’ quantity
- Estimation of the spacers’ quantity
- Estimation of the reinforcements' quantity
- Total estimation of the materials’ quantities
- Optimization of the reinforcement schedule
- Estimation of the structural frame’s cost
- Electronic exchange of designs - bids - orders
Detailing drawings
- General
- The drawings’ title block
- Carpenter’s drawings
- Steel fixer’s drawings
Tables
- Table 1
- Table 2
- Table 3
Drawings
- Carpenter’s drawings
- Steel fixer’s drawings
Model (exemplary) construction

« Effective span of beams and slabs [EC2 A§5.3.2.2] Rigid body »

Effective width of flanges [EC2 A§5.3.2.1]

[EC2 A§5.3.2.1]

Τhe effective flange width b_eff depends on the distance l_o between consecutive zero moment points and on half the spans b₁, b₂ at both sides of slabs.

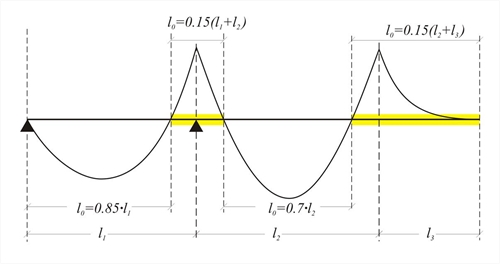Figure 3.1.2-1: Continuous beam: l_o distance between consecutive points of zero moments

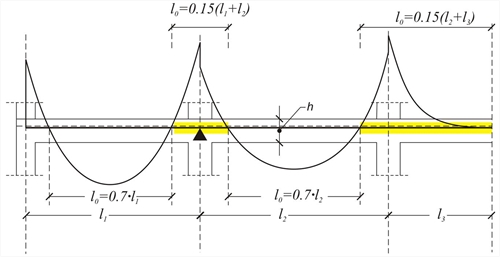Figure 3.1.2-2: Continuous beam of frames: l_o distance between consecutive points of zero moments

Earthquake resistant structures require strong columns and fixed column-beam connections. This requirement demands the creation of a frame set of beams, forming a continuous structure with respect to geometry, but autonomous with respect to the adjacent beams. This fact leads to the conclusion that, in general, the supports of a beam are rarely hinged. Therefore, l_o=0.70·l can be chosen for all the earthquake resistant beams.

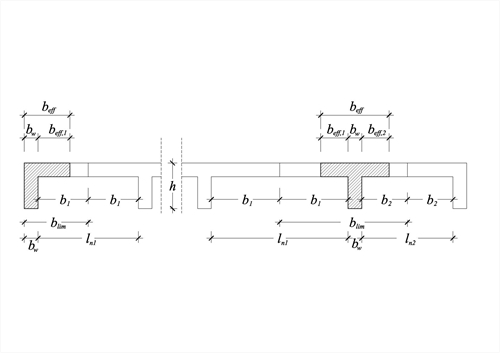Figure 3.1.2-3

b _eff =b _w +b _eff,1 +b _eff,2 ≤b _lim

where b_eff,1=0.20·b₁+0.10·l_o≤0.20·l_oandb_eff,2=0.20·b₂+0.10 ·l_o≤0.20·l_o.

Notes

Τhe effective widths at supports have practical meaning mainly for the dimensioning of inverted concrete beams under bending.
When an adjacent slab is cantilever, of a span l_n, the corresponding b₁ή b₂ is equal to l_n.

Example 3.1.2

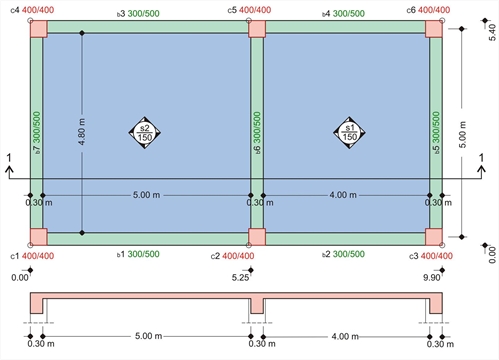Figure 3.1.2-4: Woodwork plan and section 1-1

The end beam b5 has cross-section 300/5 and span l=5.00 m. The clear span on the left of slab s1 is l_n1=4.00 m. The calculation of the effective flange width is requested.

The flange is of type Γ.

l_o=0.70·l=0.70x5.00=3.50 m

b₁=l_n1/2=4.00/2=2.00 m, b_lim=b_w+b₁=0.30+2.00=2.30 m

Τhe effective width is equal to:

b_eff=min(b_w+b_eff,1, b_lim)=min(0.30+0.70, 2.30)=1.00 m

where

b_eff,1=min(0.20·b₁+0.10·l_o,0.20·l_o)=min(0.20x2.00+0.10x3.50, 0.20x3.50)=min(0.75, 0.70)=0.70 m.

The middle beam b6 has the same dimensions as b5. The span of the slab on the left of b6 is l_n1=5.00 m. The span of the slab on the right of b6 is l_n2=4.00 m. The calculation of the effective flange width is requested.

This flange is of type T.

b₁=l_n1/2=5.00/2=2.50 m, b₂=l_n2/2=4.00/2=2.00 m, b_lim=b_w+b₁+b₂ =0.30+2.50+2.00=4.80 m

Τhe effective width is equal to:

b_eff=min(b_w+b_eff,1+b_eff,2, b_lim)=min(0.30+0.70+0.70, 4.80)=1.70 m

where

b_eff_,2=0.70 m (same with b_eff_,1 of beam b5)

b_eff,1=min(0.20·b₁+0.10·l_o,0.20·l_o )=min(0.20x2.50+0.10x3.5,0.20x3.50)=min(0.85, 0.70)=0.70 m.

« Effective span of beams and slabs [EC2 A§5.3.2.2] Rigid body »