Exercise

The residential building of the sketch includes a basement of area 12x18 m² and height 3 m, a ground floor and four storeys of identical dimensions and a top floor of area 4x6 m² and height 2.5 m. The masses at levels 0, 1, 2, 3, 4 are equal to M_G=220 t and M_Q=44 t, at level 5 to M_G=180 t and M_Q=44 t, while at the top level to M_G=20 t and M_Q =4 t.

Figure 2.5-1: The geometrical and loading model of the building's wind and earthquake actions

Figure 2.5-1: The geometrical and loading model of the building's wind and earthquake actions

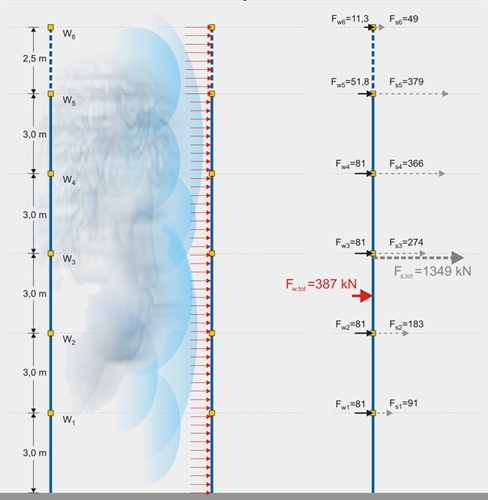Figure 2.5-2: Wind forces Fw are less significant comparing to earthquake forces Fs.

Figure 2.5-2: Wind forces Fw are less significant comparing to earthquake forces Fs.

The design acceleration at the CM is 0.12g. Therefore acceleration at the 1^st level is equal to a₁ =(3.0/9.0)x0.12g=0.04g. Respectively, at the rest levels:

a ₂ =0.08g , a₃=0.12g, a₄=0.16g, a₅=0.20g and a₆ =0.24g.

The seismic force imposed on level 'i' is:

F _s,i =M _G+0.30Q,i· a_i

therefore

F _s,0 =0

F _s,1 =233t · (0.04x9.81)m/sec ² =91 kN

F _s,2 =233t · (0.08x9.81)m/sec ² =183 kN

F _s,3 =233t · (0.12x9.81)m/sec ² =274 kN

F _s,4 =233t · (0.16x9.81)m/sec ² =366 kN

F _s,5 =193t · (0.20x9.81)m/sec ² =379 kN

F _s,6 =21t · (0.24x9.81)m/sec ² =49 kN

The total seismic force (or the total seismic base shear force) F_s,tot is equal to the sum seismic forces at different levels and its center of application is located at about 2/3 of the building height.

F _s,tot =1146t · (0.12x9.81)m/sec ² =1349 kN

Assessment of wind forces

For maximum wind load w=1.50 kN/m², the corresponding force towards the most unfavourable vertical direction of the 18.0 m on each storey is F_w=18.0m·3.0m·1.50kN/m²=81 kN,
while on the top floor is F_w=6.0m·2.5m·1.50kN/m²=23 kN.
The wind load on each storey is equally shared between the two levels defining it. Therefore,

6^th level: F_w,6=23/2=12 kN

5^th level: F_w,5=23/2+81/2=52 kN

Rest levels: F_w,i=0-4=81/2+81/2=81 kN

The total wind force is N_w,tot=12+52+4x81=388 kN and its point of application is located at about 1/2 of the building height.

Comparison of wind and seismic forces

Wind forces represent 388/1349=29% of the seismic forces and their CM is at (1/2)/(2/3)=75% of the CM of seismic forces. Consequently the seismic forces are of much greater value as well as importance than the wind forces. [*]NoteTheoretically the less significant seismic forces could result in seismic area Ζ₁ in soil type Α (S=1.0) with γ_I=1.0 and q=6.75. The interval 0.15≤T≤0.50 gives spectral acceleration S_d=γ_I*a_gR*S*2.5/q=0.16g*2.5/6.75=0.059g → F_s,tot=1146t*(0.059x9.81)m/sec²=663kN.Theoretically the most critical rectangular building plan of area 12x18=216 m² with respect to wind forces is the one with sides aspect ratio 1/4 [EC8 4.2.3.2(5)], resulting to plan dimensions 30x7.2 approximately. The wind force for the side of 30 m width, is F_w=30.0x3.0x1.50=135 kN for each storey while at the top floor remains F_w=6.0x2.5x1.50=22.5 kN. Therefore the wind load at the 6^th level is F_w,6=22.5/2=11 kN, at the 5^th level F_w,5=22.5/2+135/2=79 kN, while at the rest F_w,i=135/2+135/2=135 kN. The total wind force is F_w,tot=11+79+4x135=630 kN. .

Models Analysιs

Structural model